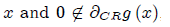On the equality of Clarke-Rockafellar and Greenberg-Pierskalla differentials for monotone and quasiconcave functionals

We study monotone, continuous, and quasiconcave functionals defifined over an M-space. We show that if g is also Clarke-Rockafellar differentiable at (see below picture) , then the closure of Greenberg- Pierskalla differentials at x coincides with the closed cone generated by the Clarke-Rockafellar differentials at x. Under the same assumptions, we show that the set of normalized Greenberg-Pierskalla differentials at x coincides with the closure of the set of normalized Clarke-Rockafellar differentials at x. As a corollary, we obtain a differential characterization of quasiconcavity a la Arrow and Enthoven (1961) for Clarke-Rockafellar differentiable functions.